Minimum Number of Steps to Make Two Strings Anagram

Last Updated on November 27, 2023 by Ankit Kochar

In the realm of string manipulation, anagrams hold a significant place due to their intriguing nature. An anagram is formed by rearranging the letters of one word or phrase to create another, using all the original letters exactly once. When it comes to making two strings anagrams of each other, the challenge lies in determining the minimum number of steps required to transform one string into another. These steps often involve altering, deleting, or adding characters within the strings to achieve the desired result. Understanding the concept of anagram transformation and the minimum steps involved provides valuable insights into string manipulation algorithms and problem-solving techniques. This article explores the intriguing puzzle of determining the minimum number of steps needed to convert one string into an anagram of another, unraveling various approaches and strategies to solve this computational challenge.

How to Find the Minimum Number of Steps to Make Two Strings Anagram?How to Find the Minimum Number of Steps to Make Two Strings Anagram?

For given two string, Print minimum number of steps to make them anagram.

See original problem statement here

Test Case

Input:
1
expertcoder zenithcoder

Output:
4

Explanation:
In 'expertcoder' and 'zenithcoder', 7 characters ('e','t','c','o','d','e','r') are common in both. 

Characters which are not common are :
In 'expertcoder' : 'e','x','p','r'
In 'zenithcoder' : 'z','n','i','h'

If we convert, 'e' to 'z', 'x' to 'n', 'p' to 'i' and 'r' to 'h', both strings becomes Anagram.

Solving Approach :

Anagram: Two strings are anagram if both of them contain the same characters with a different arrangement. For example prepbytes and bytesprep are anagrams.

1. We can count a minimum number of changes if we can find if how many numbers of characters are different in one string from another string. We do it for both of the strings.

2. We check this by scanning both strings, and increase value by 1 for the current character in 1^st string and decrease the value by 1 for the current character in 2^nd string.

3. After scanning both strings, we find the absolute sum of the hash table where sum represents the number of characters different in both strings.

4. After checking this out, we know a single change would affect two characters, if we change one character to another one which is extra in second string and missing in the first string.

5. So we print half of that sum as our answer.

Example

• Lets assume two strings to be ‘prepbuddy‘ and ‘codepuddy‘.
• As we defined in the definition of anagram, two strings must have the same number of characters in any order. So we first find the number of characters which are different in both strings.
• We can calculate such characters using a hash table.
• After scanning both strings our hash table becomes

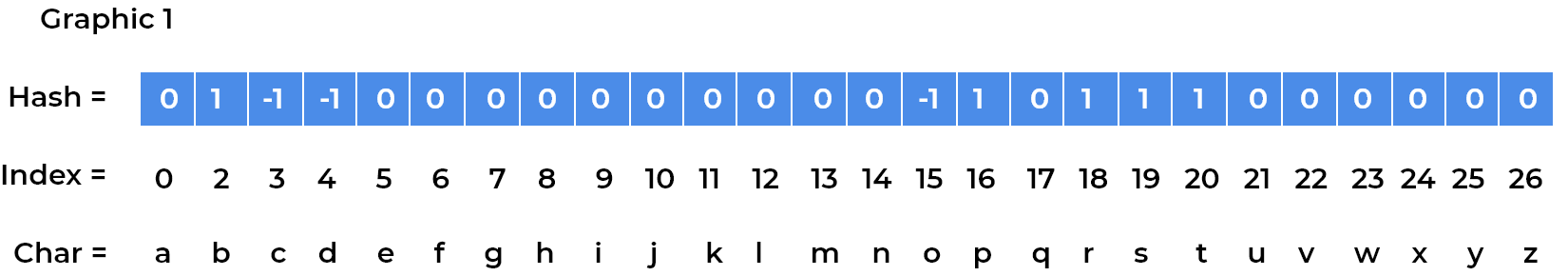
• 1 in hash table means character is present in 1^st string but not in 2^nd string, and -1 in hash table means character is present in 2^nd string but not in 1^st string,.

• Absolute sum of the hash table is 6 which means a total of 6 characters are different, half of it i.e. 3 is our final answer.

Solutions

#include <stdio.h>
    #include<string.h>
    int main()
    {
      int test;
      scanf("%d", &test);

      while(test--){

        char a[100000], b[100000];
        scanf("%s%s", a,b);

        int n = strlen(a);
        int hash[27] = {0}, count=0;

        for(int i=0; i<n; i++){
            hash[a[i]-'a']++;
            hash[b[i]-'a']--;
        }

        for(int i=0; i<26; i++){
            if(hash[i]<0)
              count -= hash[i];
        }

        printf("%d\n", count);
      }
    }

#include <bits/stdc++.h>
    using namespace std;

    int main()
    {
      int test;
      cin>>test;

      while(test--){

        string a, b;
        cin>>a>>b;

        int hash[26] = {0}, count=0;
        for(int i=0; i<b.length(); i++){
            hash[a[i]-'a']++;
            hash[b[i]-'a']--;
        }

        for(int i=0; i<26; i++){
            if(hash[i]<0)
              count -= hash[i];
        }

        cout<<count<<endl;
      }
    }

import java.util.*;
    import java.io.*;
    import java.lang.Math;

    public class Main {
      public static void main(String args[]) throws IOException {

        Scanner sc= new Scanner(System.in);
        int test = sc.nextInt();
          while(test != 0){

            String a = sc.next();
            String b = sc.next();

            int hash[] = new int[26];
            int count=0;

            for(int i=0; i<b.length(); i++){
                hash[a.charAt(i)-'a']++;
                hash[b.charAt(i)-'a']--;
            }

            for(int i=0; i<26; i++){
                if(hash[i]<0)
                  count -= hash[i];
            }

            System.out.println(count);
            test--;
          }

      }

for _ in range(int(input())):
	
	a, b = input().split()
	hash = [0] * 26
	count = 0
	
	for i in range(len(b)):
		hash[ord(a[i]) - ord("a")] += 1
		hash[ord(b[i]) - ord("a")] -= 1

	for i in range(26):
		if hash[i] < 0:
			count -= hash[i]

	print(count)

Conclusion:
In conclusion, the minimum number of steps required to make two strings anagrams of each other unveils an engaging puzzle in the realm of string manipulation and algorithmic problem-solving. Through this exploration, we’ve delved into diverse methodologies and techniques to decipher this challenge, from frequency-based approaches to leveraging different algorithms. Understanding the intricacies of anagram transformations not only sheds light on fundamental string manipulation concepts but also enhances problem-solving skills in computer science and programming. As we continue to explore the fascinating world of strings and their transformations, the quest for efficient algorithms to determine the minimum steps for anagram conversion remains an ongoing and captivating pursuit.

FAQ Related to Minimum Number of Steps to Make two Strings Anagram:

Here are some FAQs related to Minimum Number of Steps to Make Two Strings Anagram.

1. What is an anagram?
An anagram is a word or phrase formed by rearranging the letters of another word or phrase, using all the original letters exactly once. For example, "listen" and "silent" are anagrams of each other.

2. How do you determine the minimum steps to make two strings anagrams?
The minimum number of steps required to make two strings anagrams involves comparing their characters and calculating the differences in their frequencies. By tallying the count of characters in each string and identifying the discrepancies, one can determine the minimum alterations needed to transform one string into an anagram of the other.

3. Can you provide an example of determining the minimum steps for anagram conversion?
Sure! Consider two strings, "abcde" and "abbcf." To make "abcde" an anagram of "abbcf," you’d need to change the second string to "abcde" by adding an ‘e’ and removing an extra ‘b’ and ‘f.’ Therefore, the minimum number of steps required is adding one character and removing two characters.